For a reaction, 2A + B → C + D, the following observations were recorded:

Experiment	[A]/mol L–1	[B]/mol L–1	Initial rate of formation of D/mol L–1 min–1
I	0.1	0.1	6.0 × 10–3
II	0.3	0.2	7.2 × 10–2
III	0.3	0.4	2.88 × 10–1
IV	0.4	0.1	2.40 × 10–2

The rate law applicable to the above mentioned reaction would be:

1. Rate = k[A]²[B]³

2. Rate = k[A][B]²

3. Rate = k[A]²[B]  

4. Rate = k[A][B]  

Given the following observations:

The reaction between A and B is first-order with respect to A and zero-order with respect to B. The values of X and Y are, respectively:

1.  X = 0.2 \(mol\) \(L^{- 1}\); Y = \(\) \(0 . 08\) \(mol\) \(L^{- 1} \left(min\right)^{- 1}\)

2.  X = 0.02 \(mol\) \(L^{- 1}\); Y = \(\) \(0 . 08\) \(mol\) \(L^{- 1} \left(min\right)^{- 1}\)

3.  X = 0.01 \(mol\) \(L^{- 1}\); Y = \(\) \(0 . 8\) \(mol\) \(L^{- 1} \left(min\right)^{- 1}\)

4. X = 0.2 \(mol\) \(L^{- 1}\); Y = \(\) \(0 . 8\) \(mol\) \(L^{- 1} \left(min\right)^{- 1}\)

A radioactive substance has a rate constant of \(4 \, \text{years}^{-1}\). What is its half-life?

1. 0.05 years

2. 0.17 years

3. 0.26 ${\mathrm{years}}^{-1}$

4. 1.6 years

During a nuclear explosion, one of the products is ⁹⁰Sr with a half-life of 28.1 years. If 1µg of ⁹⁰Sr was absorbed in the bones of a newly born baby instead of calcium, the amount of ⁹⁰Sr that will remain after 10 years in the now grown up child would be -

(Given ,antilog(0.108)=1.28)

1. 0.227 µg 

2. 0.781 µg 

3. 7.81 µg 

4. 2.27 µg 

For the decomposition of azoisopropane to hexane and nitrogen at 543 K, the following data was obtained:

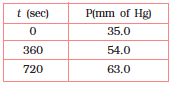
The rate constant of the above reaction would be -

For the  reaction $2\mathrm{A} + \mathrm{B}\to {\mathrm{A}}_2\mathrm{B}$, rate = $\mathrm{k}\left[\mathrm{A}\right]{\left[\mathrm{B}\right]}^2$ with k = $2.0\times {10}^{-6} {\mathrm{mol}}^{-2}{\mathrm{L}}^2{\mathrm{s}}^{-1}$ and $\left[\mathrm{A}\right]=0.1 \mathrm{M}; \left[\mathrm{B}\right]=0.2 \mathrm{M}$. The initial rate of the reaction will be :

1. 0.04$\times {10}^{-9}$ mol ${\mathrm{L}}^{-1} {\mathrm{s}}^{-1}$

2. 8$\times {10}^{-8} {\mathrm{mol}}^{-1}\mathrm{L} {\mathrm{s}}^{-1}$

3. 8$\times {10}^{-9} \mathrm{mol} {\mathrm{L}}^{-1} {\mathrm{s}}^{-1}$

4. 8$\times {10}^{-7}$ mol ${\mathrm{L}}^{-1} {\mathrm{s}}^{-1}$

For a first-order reaction, the relationship between time required for 99% completion to the time required for the completion of 90% of the reaction would be :

What are the dimensions of the rate constant K in the rate law \(\text { Rate }=k\left[H_2 O_2\right]\left[I^{-}\right]\)?
 

Consider the following rate expression.

$\mathrm{Rate}=\mathrm{k}{\left[C{H}_{3}CHO\right]}^{\frac{3}{2}}$

The order of reaction and dimension of the rate constant are, respectively-

1. $\raisebox{1ex}{$3$}\!\left/ \!\raisebox{-1ex}{$2$}\right.$; k = ${\mathrm{mol}}^{-1}{\mathrm{Ls}}^{-1}$

2. 3; k = ${\mathrm{mol}}^{-1}{\mathrm{Ls}}^{-1}$

3. $\raisebox{1ex}{$3$}\!\left/ \!\raisebox{-1ex}{$2$}\right.$;  k = ${\mathrm{mol}}^{\frac{1}{2}}{\mathrm{L}}^{-\frac{1}{2}}{\mathrm{s}}^{-1}$

4. $\raisebox{1ex}{$3$}\!\left/ \!\raisebox{-1ex}{$2$}\right.$; k = ${\mathrm{mol}}^{-\frac{1}{2}}{\mathrm{L}}^{\frac{1}{2}}{\mathrm{s}}^{-1}$

If the concentration of the reactant is made twice, the new rate of reaction for the second order reaction would be-

1. 2 times

2. 4 times

3. 3 times

4. No change in the rate of the reaction