In the circuit of figure, what will be the reading of the voltmeter?
(1) 300 V (2) 900 V
(3) 200 V (4) 400 V
In a LCR circuit capacitance is changed from C to 2C. For the resonant frequency to remain unchanged, the inductance should be changed from L to
(1) 4 L (2) 2 L
(3) L/2 (4) L/4
In a series R-L-C AC circuit, for a particular value of R, L and C, power supplied by the source is P at resonance. If the value of inductance is halved, then the power from the source again at resonance is P'. Then
(1) P =
(2) P = 2P'
(3) P = 4P'
(4) P = P'
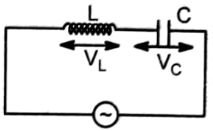
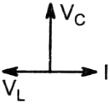
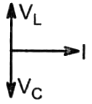
The current I, potential difference VL across the inductor and potential difference VC across the capacitor in the circuit as shown in the figure are best represented vectorially as:
1. 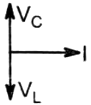 2.
2. 
3. 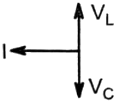 4.
4. 
A 120 volt AC source is connected across a pure inductor of inductance 0.70 Henry. If the frequency of the source is 60 Hz, the current passing through the inductor is :
(1) 4.55 A (2) 0.325 A
(3) 0.455 A (4) 3.25 A
A series combination of R, L, C is connected to an a.c. source. If the resistance is 3 and the reactance is 4 , the power factor of the circuit is
(1) 0.4 (2) 0.6 (3) 0.8 (4) 1.0
The voltage and current in a series AC circuit are given by V = cos What is the power dissipated in the circuit?
(1) (2) 0 (3) (4)
A coil has an inductance of 0.7 H and is joined in series with a resistance of 220 . An alternating emf of 220 V at 50 Hz is applied to it. Then the wattless component of the current in the circuit is
(1) 5 amp (2) 0.5 amp (3) 0.7 amp (4) 7 amp
Resonance occurs in a series L-C-R circuit when the frequency of the applied e.m.f. is 1000 Hz. Then:
1. when f=900 Hz, the circuit behaves as a capacitive circuit
2. the impendence of the circuit is maximum at f=1000 Hz
3. at resonance the voltages across L and current in C differ in phase by 180
4. if the value of C is doubled resonance occurs at f=2000 Hz
The root-mean-square value of an alternating current of \(50~\text{Hz}\) frequency is \(10 ~\text A\). The time taken by the alternating current to reach from zero to maximum value and peak value will be:
1. \(2×10^{–2}~\text s\) and \(14.14 ~\text A \)
2. \(2×10^{–2}~\text s\) and \(7.07~\text A\)
3. \(5×10^{–3}~\text s\) and \(7.07~\text A\)
4. \(5×10^{–3}~\text s\) and \(14.14 ~\text A \)







