Q. No.A metallic spherical shell has an inner radius \(R_1\) and an outer radius \(R_2.\) A point charge \(Q\) is placed at the center of the spherical cavity. What are the surface charge densities \(\sigma_{in}\) and \(\sigma_{out}\) on the inner and outer surfaces of the shell, respectively?
1. \(\sigma_{in} = -\dfrac{Q}{4\pi R_1^2},~ \sigma_{out}=\dfrac{Q}{4\pi R_2^2}\)
2. \(\sigma_{in} = \dfrac{Q}{4\pi R_1^2},~ \sigma_{out}=0\)
3. \(\sigma_{in} = 0,~ \sigma_{out}=\dfrac{Q}{4\pi R_2^2}\)
4. \(\sigma_{in} = \dfrac{Q}{4\pi R_1^2},~ \sigma_{out}=-\dfrac{Q}{4\pi R_2^2}\)
1. \(\sigma_{in} = -\dfrac{Q}{4\pi R_1^2},~ \sigma_{out}=\dfrac{Q}{4\pi R_2^2}\)
2. \(\sigma_{in} = \dfrac{Q}{4\pi R_1^2},~ \sigma_{out}=0\)
3. \(\sigma_{in} = 0,~ \sigma_{out}=\dfrac{Q}{4\pi R_2^2}\)
4. \(\sigma_{in} = \dfrac{Q}{4\pi R_1^2},~ \sigma_{out}=-\dfrac{Q}{4\pi R_2^2}\)
| 1. |  |
| 2. |  |
| 3. |  |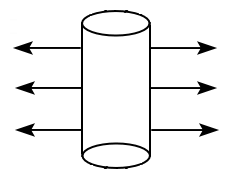
| 4. |  |
What will be the total flux through the faces of the cube as given in the figure with a side of length 'a' if a charge q is placed at?
Match the following:
| Column I | Column II | ||
| (a) | a corner of the cube | (i) | \(\phi=\dfrac{q}{2\epsilon_0}\) |
| (b) | mid-point of an edge of the cube | (ii) | \(\phi=\dfrac{q}{8\epsilon_0}\) |
| (c) | centre of a face of the cube | (iii) | \(\phi=\dfrac{q}{2\epsilon_0}\) |
| (d) | mid-point of B and C | (iv) | \(\phi=\dfrac{q}{4\epsilon_0}\) |
| 1. | (A)\(\rightarrow \)(iv), (B)\(\rightarrow \)(ii), (C)\(\rightarrow \)(iii), (D)\(\rightarrow \)(i) |
| 2. | (A)\(\rightarrow \)(ii), (B)\(\rightarrow \)(iv), (C)\(\rightarrow \)(i), (D)\(\rightarrow \)(iii) |
| 3. | (A)\(\rightarrow \)(iii), (B)\(\rightarrow \)(ii), (C)\(\rightarrow \)(iv), (D)\(\rightarrow \)(i) |
| 4. | (A)\(\rightarrow \)(i), (B)\(\rightarrow \)(iii), (C)\(\rightarrow \)(ii), (D)\(\rightarrow \)(iv) |
The figure represents a crystal unit of caesium chloride, CsCl. The caesium atoms, represented by open circles are situated at the corners of a cube of side 0.40 nm, whereas a Cl-atom is situated at the centre of the cube. The Cs-atoms are deficient in one electron while the Cl-atom carries an excess electron. What is the net electric field \(E_{net}\) on the Cl-atom due to eight Cs-atoms, and suppose that the Cs-atom at corner A is missing. What is the net force \(F_{net}\) now on the Cl-atom due to seven remaining Cs-atoms?
| 1. | \(E_{net}=1.2 \times 10^9 ~\text{NC}^{-1},~F_{net} = 1.92 \times 10^{-9}~\text N\) |
| 2. | \(E_{net}=0~\text{NC}^{-1},~F_{net} = 1.92 \times 10^{-9}~\text N\) |
| 3. | \(E_{net}=0~\text{NC}^{-1},~F_{net} = 0~\text N\) |
| 4. | \(E_{net}=1.2\times 10^9~\text{NC}^{-1},~F_{net} = 0~\text N\) |
Two charges q and -3q are placed fixed on x-axis separated by distance d. Where should a third charge 2q be placed such that it will not experience any force?
| 1. | At a distance \(\dfrac{d}{\sqrt3 -1}\) to the left of charge \(q\) |
| 2. | At a distance \(\dfrac{d(\sqrt3 -1)}2\) to the left of charge \(q\) |
| 3. | At a distance \(\dfrac{d}{2}\) to the right of charge \(-3q\) |
| 4. | Midway between \(q\) and \(-3q\) |
1. \(\phi_E =\dfrac{q}{\epsilon_0}\)
2. \(\phi_E =\dfrac{2q}{\epsilon_0}\)
3. \(\phi_E =\dfrac{p}{\epsilon_0 r^2}\)
4. \(\phi_E = 0\)
| (a) | if \(q>0\) and is displaced away from the centre in the plane of the ring, it will be pushed back towards the centre. |
| (b) | if \(q<0\) and is displaced away from the centre in the plane of the ring, it will never return to the centre and will continue moving till it hits the ring. |
| (c) | if \(q<0\), it will perform SHM for small displacement along the axis. |
| (d) | q at the centre of the ring is in an unstable equilibrium within the plane of the ring for \(q>0\). |
1. (a), (b), (c)
2. (a), (c), (d)
3. (b), (c), (d)
4. (c), (d)
Refer to the arrangement of charges in the figure and a Gaussian surface of a radius \(R\) with \(Q\) at the centre. Then:
| (a) | total flux through the surface of the sphere is \(\frac{-Q}{\varepsilon_0}.\) |
| (b) | field on the surface of the sphere is \(\frac{-Q}{4\pi \varepsilon_0 R^2}.\) |
| (c) | flux through the surface of the sphere due to \(5Q\) is zero. |
| (d) | field on the surface of the sphere due to \(-2Q\) is the same everywhere. |
Choose the correct statement(s):
| 1. | (a) and (d) | 2. | (a) and (c) |
| 3. | (b) and (d) | 4. | (c) and (d) |
| (a) | the electric field is necessarily zero. |
| (b) | the electric field is due to the dipole moment of the charge distribution only. |
| (c) | the dominant electric field is \(\propto \dfrac 1 {r^3}\), for large \(r\), where \(r\) is the distance from the origin in this region. |
| (d) | the work done to move a charged particle along a closed path, away from the region, will be zero. |
Which of the above statements are true?
1. (b) and (d)
2. (a) and (c)
3. (b) and (c)
4. (c) and (d)
| (a) | \(\oint_s {E} . {dS} \neq 0\) on any surface |
| (b) | \(\oint_s {E} . {dS} = 0\) if the charge is outside the surface. |
| (c) | \(\oint_s {E} . {dS}\) could not be defined. |
| (d) | \(\oint_s {E} . {dS}=\frac{q}{\epsilon_0}\) if charges of magnitude \(q\) were inside the surface. |
| 1. | (a) and (d) | 2. | (a) and (c) |
| 3. | (b) and (d) | 4. | (c) and (d) |
© 2025 GoodEd Technologies Pvt. Ltd.