A particle is dropped from a height of 50 m. If the particle loses its 20% mechanical energy during the impact with the ground, up to what height will it rebound after the second impact?

(1)  40 m

(2)  36 m

(3)  32 m

(4)  28 m

A body of mass 2 kg is rotating in a vertical circle of radius 4 m. The difference in its kinetic energy at the top and bottom of the circle is:

(1)  40 J

(2)  80 J

(3)  120

(4)  160 J

If a stone is projected vertically upward from the ground with a speed of \(10~\text{m/s}\), then its:
(take \(g=10\) m/s²)

A body of mass m strikes with the ground with speed u and coefficient of restitution is e, then find the work done by normal reaction force on the body.

1.  zero

2.  $\frac{1}{2}{\mathrm{mu}}^2\left({\mathrm{e}}^2 - 1\right)$

3.  $\frac{1}{2}{\mathrm{mu}}^2\left(1 - {\mathrm{e}}^2\right)$

4.  $\frac{1}{2}{\mathrm{mu}}^2$

A particle suspended by a light inextensible thread of length l is projected horizontally from its lowest position with velocity $\sqrt{4gl}$. The height from its lowest position at which particle will leave the circular path is:

1.  $\frac{3\mathrm{l}}{2}$

2.  $\frac{5l}{3}$

3.  $\frac{4l}{3}$

4.  2l

A mass M is suspended by a spring having a spring constant K. In equilibrium position mass M is given a speed u. Find further extension in the spring.

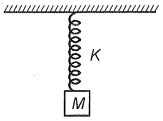
1.  $\sqrt{\frac{\mathrm{M}}{\mathrm{K}}}\mathrm{u}$

2.  $\sqrt{\frac{2\mathrm{M}}{\mathrm{K}}}\mathrm{u}$

3.  $\sqrt{\frac{\mathrm{M}}{\mathrm{K}}}\mathrm{u} + \frac{\mathrm{Mg}}{\mathrm{K}}$

3.  $\sqrt{\frac{2\mathrm{M}}{\mathrm{K}}}\mathrm{u} + \frac{\mathrm{Mg}}{\mathrm{K}}$

A ball 'P strikes elastically with another identical ball Q resting on a smooth surface with velocity v. The ratio of the speeds of ${\mathrm{v}}_{\mathrm{P}} : {\mathrm{v}}_{\mathrm{e}}$ two balls after the collision is

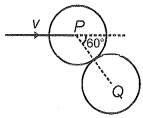
(1)  1: $\sqrt{3}$

(2)  $\sqrt{3}$:1

(3)  1:2

(4)  $\sqrt{3}$:2

A block of mass 20 kg is being brought down by a chain. If block acquires a speed of 2 m/s in dropping down 2 m. Find work done by the chain during the process. (g = 10 $\mathrm{m}/{\mathrm{s}}^2$)

(1)  -360 J

(2)  400 J

(3)  360 J

(4)  -280 J

A ball is dropped from a height h on a stationary floor and rebounds. If the coefficient of restitution is 0.5, then the total distance covered by the ball before it strikes the floor for 3rd time is:

1.  2h

2.  $\frac{13\mathrm{h}}{8}$

3.  $\frac{3\mathrm{h}}{2}$

4.  $\frac{4\mathrm{h}}{3}$

A particle is projected at a time \(t=0\) with a speed \(v_{0}\)${\mathrm{}}_{}$ and at an angle with the horizontal in a uniform gravitational field. Then which of the following graphs represents power delivered by the gravitational force against time \((t)?\)

1.		2.
3.		4.