A ball is thrown vertically downwards with a velocity of \(20\) m/s from the top of a tower. It hits the ground after some time with the velocity of \(80\) m/s . The height of the tower is: (assuming \(g = 10~\text{m/s}^2)\)
1.
\(340\) m
2.
\(320\) m
3.
\(300\) m
4.
\(360\) m
A particle starts from rest (with constant acceleration) and acquires velocity \(20\) m/s in \(5\) s. The distance travelled by the particle in the next \(2\) s will be:
| 1. | \(50\) m | 2. | \(48\) m |
| 3. | \(100\) m | 4. | \(150\) m |
A drunkard walking in a narrow lane takes \(5\) steps forward and \(3\) steps backward, followed again by \(5\) steps forward and \(3\) steps backward, and so on. Each step is \(1\) m long and requires \(1\) s. There is a pit on the road \(13\) m away from the starting point. The drunkard will fall into the pit after:
1. \(37\) s
2. \(31\) s
3. \(29\) s
4. \(33\) s
The figure gives the \((x\text-t)\) plot of a particle in a one-dimensional motion. Three different equal intervals of time are shown. The signs of average velocity for each of the intervals \(1,\) \(2\) and \(3,\) respectively are:

| 1. | \(-,-,+\) | 2. | \(+,-,+\) |
| 3. | \(-,+,+\) | 4. | \(+,+,-\) |
| 1. | zero velocity. | 2. | zero acceleration. |
| 3. | non-zero velocity. | 4. | non-zero acceleration. |
When the velocity of a body is variable, then:
| 1. | its speed may be constant |
| 2. | its acceleration may be constant |
| 3. | its average acceleration may be constant |
| 4. | all of the above |
A body is projected vertically in the upward direction from the surface of the earth. If the upward direction is taken as positive, then the acceleration of the body during its upward and downward journey is:
| 1. | Positive, negative | 2. | Negative, negative |
| 3. | Positive, positive | 4. | Negative, positive |
Which one of the following displacement-time graph represents two moving objects \(P\) and \(Q\) with zero relative velocity?
| 1. | 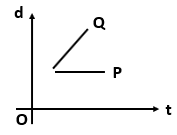 |
2. | 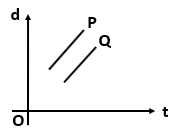 |
| 3. | 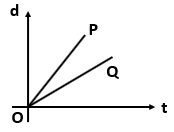 |
4. | 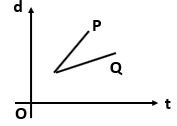 |
Suppose you are riding a bike with a speed of \(20~\text{m/s}\) due east relative to a person \(A\) who is walking on the ground towards the east. If your friend \(B\) walking on the ground due west measures your speed as \(30~\text{m/s}\) due east, then the relative velocity between two reference frames \(A\) and \(B\) is:
| 1. | the velocity of \(A\) with respect to \(B\) is \(5~\text{m/s}\) towards the east. |
| 2. | the velocity of \(A\) with respect to \(B\) is \(5~\text{m/s}\) towards the west. |
| 3. | the velocity of \(A\) with respect to \(B\) is \(10~\text{m/s}\) towards the east. |
| 4. | the velocity of \(A\) with respect to \(B\) is \(10~\text{m/s}\) towards the west. |
A particle is moving along the \(x\)-axis such that its velocity varies with time as per the equation \(v = 20\left(1-\frac{t}{2}\right) \). At \(t=0 \) particle is at the origin. From the following, select the correct position \((x)\) - time \((t)\) plot for the particle:
| 1. |  |
2. |  |
| 3. |  |
4. |  |




