A player throws a ball upwards with an initial speed of \(29.4 \text{ ms}^{- 1}.\) What is the direction of acceleration during the upward motion of the ball?
1. Vertically downwards
2. Vertically upwards
3. First upwards then downwards
4. None of the above
1. Vertically downwards
2. Vertically upwards
3. First upwards then downwards
4. None of the above
A particle in one-dimensional motion:
| 1. | with zero speed at an instant may have non-zero acceleration at that instant. |
| 2. | with zero speed may have non-zero velocity. |
| 3. | with constant speed, must have non-zero acceleration. |
| 4. | with a positive value of acceleration must be speeding up. |
A man walks on a straight road from his home to a market \(2.5\) km away with a speed of \(5\) km/h. Finding the market closed, he instantly turns and walks back home with a speed of \(7.5\) km/h. What is the magnitude of the average velocity of the man over the interval of time \(0\) to \(30\) min?
| 1. | \(6\) km/h | 2. | \(5\) km/h |
| 3. | \(5.6\) km/h | 4. | \(6.6\) km/h |
The instantaneous speed is always:
| 1. | less than the magnitude of instantaneous velocity. |
| 2. | greater than the magnitude of instantaneous velocity. |
| 3. | equal to the magnitude of instantaneous velocity. |
| 4. | may be less or greater than the magnitude of instantaneous velocity. |
A police van moving on a highway with a speed of fires a bullet at a thief’s car speeding away in the same direction with a speed of . If the muzzle speed of the bullet is with what speed does the bullet hit the thief’s car?
The figure gives the \((x\text-t)\) plot of a particle in a one-dimensional motion. Three different equal intervals of time are shown. The signs of average velocity for each of the intervals \(1,\) \(2\) and \(3,\) respectively are:

| 1. | \(-,-,+\) | 2. | \(+,-,+\) |
| 3. | \(-,+,+\) | 4. | \(+,+,-\) |
The figure gives a speed-time graph of a particle in motion along the same direction. Three equal intervals of time are shown. In which interval is the average acceleration greatest in magnitude?
| 1. | Interval 2 | 2. | Interval 1 |
| 3. | Interval 3 | 4. | Equal in all intervals |
A boy standing on a stationary lift (open from above) throws a ball upwards with the maximum initial speed he can, equal to \(49~\text{ms}^{-1}.\) How much time does the ball take to return to his hands?
| 1. | \(5\) s | 2. | \(10\) s |
| 3. | \(15\) s | 4. | \(7\) s |
On a long horizontally moving belt (as shown in the figure) a child runs to and fro with a speed (with respect to the belt) between his father and mother located 50 m apart on the moving belt. The belt moves with a speed of . For an observer on a stationary platform outside, what is the speed of the child running in the direction of motion of the belt?
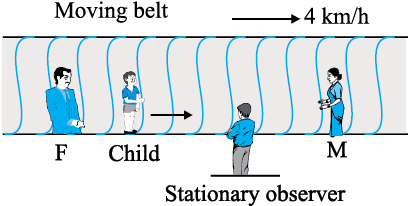
1. 5 kmh-1
2. \(\sqrt{41}\) kmh-1
3. 21 kmh-1
4. 13 kmh-1
A passenger arriving in a new town wishes to go from the station to a hotel located \(10~\text{km}\) away on a straight road from the station. A dishonest cabman takes him along a circuitous path \(23~\text{km}\) long and reaches the hotel in \(28~\text{min}.\) The average speed of the taxi is:
1. \(30~\text{km/h}\)
2. \(49.3~\text{km/h}\)
3. \(55.6~\text{km/h}\)
4. \(60~\text{km/h}\)







