- 1(current)
Q. No.| 1. | \(2\sqrt{A}\) | 2. | \(\dfrac{A}{2}\) |
| 3. | \(\dfrac{\mathrm{A}}{\sqrt{2}}\) | 4. | \(A\sqrt{2}\) |
During simple harmonic motion of a body, the energy at the extreme position is:
| 1. | both kinetic and potential |
| 2. | is always zero |
| 3. | purely kinetic |
| 4. | purely potential |
| List-I (\(x \text{-}y\) graphs) |
List-II (Situations) |
||
| (a) |  |
(i) | Total mechanical energy is conserved |
| (b) |  |
(ii) | Bob of a pendulum is oscillating under negligible air friction |
| (c) |  |
(iii) | Restoring force of a spring |
| (d) |  |
(iv) | Bob of a pendulum is oscillating along with air friction |
Choose the correct answer from the options given below:
| (a) | (b) | (c) | (d) | |
| 1. | (iv) | (ii) | (iii) | (i) |
| 2. | (iv) | (iii) | (ii) | (i) |
| 3. | (i) | (iv) | (iii) | (ii) |
| 4. | (iii) | (ii) | (i) | (iv) |
| 1. | \(3n\) | 2. | \(4n\) |
| 3. | \(n\) | 4. | \(2n\) |
A particle of mass \(m\) is released from rest and follows a parabolic path as shown. Assuming that the displacement of the mass from the origin is small, which graph correctly depicts the position of the particle as a function of time?
| 1. |  |
2. |  |
| 3. |  |
4. |  |
1. \(0~\text{and}~2K_0\)
2. \(\frac{K_0}{2}~\text{and}~K_0\)
3. \(K_0~\text{and}~2K_0\)
4. \(K_0~\text{and}~K_0\)
A particle of mass \(m\) oscillates with simple harmonic motion between points \(x_1\) and \(x_2\), the equilibrium position being \(O\). Its potential energy is plotted. It will be as given below in the graph:
| 1. |  |
2. |  |
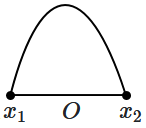| 3. |  |
4. |  |
The potential energy of a simple harmonic oscillator, when the particle is halfway to its endpoint, will be:
1. \(\frac{2E}{3}\)
2. \(\frac{E}{8}\)
3. \(\frac{E}{4}\)
4. \(\frac{E}{2}\)
1. \(\pm \frac{a}{2}\)
2. \(+a\)
3. \(\pm a\)
4. \(-1\)
The total energy of the particle performing SHM depends on:
1. \(k,\) \(a,\) \(m\)
2. \(k,\) \(a\)
3. \(k,\) \(a\), \(x \)
4. \(k,\) \(x \)
- 1(current)
Q. No.
© 2025 GoodEd Technologies Pvt. Ltd.