Select Chapter Topics:
Q. No.A particle executes SHM along a straight line.
Statement I:
A graph of its acceleration vs displacement (from mean position) is a straight line.
Statement II:
A graph of its velocity vs displacement (from mean position) is an ellipse.
1.
Statement I is incorrect and Statement II is correct.
2.
Both Statement I and Statement II are correct.
3.
Both Statement I and Statement II are incorrect.
4.
Statement I is correct and Statement II is incorrect.
Subtopic: Simple Harmonic Motion |
71%
From NCERT
Please attempt this question first.
Hints
Please attempt this question first.
Match the types of motion in List-I with their corresponding examples in List-II.
Codes:
| List-I | List-II |
| (a) motion with constant speed | (i) SHM |
| (b) motion with constant acceleration | (ii) uniform circular motion |
| (c) oscillatory motion | (iii) projectile motion |
| (d) random motion | (iv) molecular motion in gas |
Codes:
| 1. | a - (iv), b - (ii), c - (iii), d - (i) |
| 2. | a - (i), b - (iii), c - (ii), d - (iv) |
| 3. | a - (ii), b - (iii), c - (i), d - (iv) |
| 4. | a - (ii), b - (iii), c - (iv), d - (i) |
Subtopic: Simple Harmonic Motion |
91%
From NCERT
Please attempt this question first.
Hints
Please attempt this question first.
Given below are two statements:
| Statement I: | If the acceleration of a particle is directed towards a fixed point, and proportional to the distance from that point – the motion is SHM. |
| Statement II: | During SHM, the kinetic energy of the particle oscillates at twice the frequency of the SHM. |
| 1. | Statement I is incorrect and Statement II is correct. |
| 2. | Both Statement I and Statement II are correct. |
| 3. | Both Statement I and Statement II are incorrect. |
| 4. | Statement I is correct and Statement II is incorrect. |
Subtopic: Energy of SHM |
From NCERT
Please attempt this question first.
Hints
Please attempt this question first.
Given below are two statements:
| Assertion (A): | The graph between velocity and displacement for a simple harmonic motion is an ellipse. |
| Reason (R): | Velocity does not change uniformly with displacement in simple harmonic motion. |
| 1. | Both (A) and (R) are True and (R) is the correct explanation of (A). |
| 2. | Both (A) and (R) are True but (R) is not the correct explanation of (A). |
| 3. | (A) is True but (R) is False. |
| 4. | Both (A) and (R) are False. |
Subtopic: Simple Harmonic Motion |
From NCERT
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Hints
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Given below are two statements:
| Assertion (A): | Water in a \(U\text-\)tube executes SHM. The time period for mercury-filled up to the same height in the \(U\text-\)tube is greater than that in the case of water. |
| Reason (R): | The amplitude of an oscillating pendulum goes on increasing. |
| 1. | Both (A) and (R) are True and (R) is the correct explanation of (A). |
| 2. | Both (A) and (R) are True but (R) is not the correct explanation of (A). |
| 3. | (A) is True but (R) is False. |
| 4. | Both (A) and (R) are False. |
Subtopic: Simple Harmonic Motion |
52%
From NCERT
Please attempt this question first.
Hints
Please attempt this question first.
Given below are two statements:
| Assertion (A): | If the bob of a simple pendulum is kept in a horizontal electric field, its period of oscillation will remain the same. |
| Reason (R): | If bob is charged and kept in a horizontal electric field, then the time period will be decreased. |
| 1. | Both (A) and (R) are True and (R) is the correct explanation of (A). |
| 2. | Both (A) and (R) are True but (R) is not the correct explanation of (A). |
| 3. | (A) is True but (R) is False. |
| 4. | (A) is False but (R) is True. |
Subtopic: Angular SHM |
55%
From NCERT
Please attempt this question first.
Hints
Please attempt this question first.
A particle of mass \(m\) oscillates in simple harmonic motion between points \(X_{1}\) and \(X_{2},\) the equilibrium position being \(O.\) Its kinetic energy is correctly represented by which of the following graphs?
| 1. |  |
2. |  |
| 3. |  |
4. |  |
Subtopic: Energy of SHM |
76%
From NCERT
Please attempt this question first.
Hints
Please attempt this question first.
A body oscillates with SHM according to the equation (in SI units), \(x= 5\cos\left[2\pi t +\frac{\pi}{4}\right].\) At \(t = 1.5\) s, acceleration of the body will be:
| 1. | \(140 \text{ cm} / \text{s}^2 \) | 2. | \(160 \text{ m} / \text{s}^2 \) |
| 3. | \(140 \text{ m} / \text{s}^2 \) | 4. | \(14 \text{ m} / \text{s}^2\) |
Subtopic: Linear SHM |
58%
From NCERT
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Hints
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
The potential energy of a simple harmonic oscillator, when the particle is halfway to its endpoint, will be:
1. \(\frac{2E}{3}\)
2. \(\frac{E}{8}\)
3. \(\frac{E}{4}\)
4. \(\frac{E}{2}\)
Subtopic: Energy of SHM |
80%
From NCERT
AIPMT - 2003
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Hints
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
A particle of mass \(m\) oscillates with simple harmonic motion between points \(x_1\) and \(x_2\), the equilibrium position being \(O\). Its potential energy is plotted. It will be as given below in the graph:
| 1. |  |
2. |  |
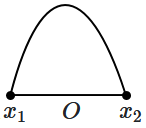| 3. |  |
4. |  |
Subtopic: Energy of SHM |
84%
From NCERT
AIPMT - 2003
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Hints
To view explanation, please take trial in the course.
NEET 2025 - Target Batch
Select Chapter Topics:
© 2024 GoodEd Technologies Pvt. Ltd.