Q. No.In a simple harmonic oscillation, the graph of acceleration against displacement for one complete oscillation will be:
1. an ellipse
2. a circle
3. a parabola
4. a straight line
1. an ellipse
2. a circle
3. a parabola
4. a straight line
\(B\), it returns to \(B\) after another \(3~\text{s}\). The time period of the SHM will be:
| 1. | \(15~\text{s}\) | 2. | \(6~\text{s}\) |
| 3. | \(12~\text{s}\) | 4. | \(9~\text{s}\) |
| 1. | The rotation of the earth about its axis. |
| 2. | The motion of an oscillating mercury column in a \(U\text-\)tube. |
| 3. | General vibrations of a polyatomic molecule about its equilibrium position. |
| 4. | A fan rotating with a constant angular velocity. |
Which of the following relationships between the acceleration \(a\) and the displacement \(x\) of a particle involves simple harmonic motion?
1. \(a = 0 . 7 x\)
2. \(a = - 200 x^{2} \)
3. \(a = - 10 x\)
4. \(a = 100 x^{3}\)
A spring having a spring constant of \(1200\) N/m is mounted on a horizontal table as shown in the figure. A mass of \(3\) kg is attached to the free end of the spring. The mass is then pulled sideways to a distance of \(2.0\) cm and released. The frequency of oscillations will be:
| 1. | \(3.0~\text{s}^{-1}\) | 2. | \(2.7~\text{s}^{-1}\) |
| 3. | \(1.2~\text{s}^{-1}\) | 4. | \(3.2~\text{s}^{-1}\) |
Identify the correct definition:
| 1. | If after every certain interval of time, a particle repeats its motion, then the motion is called periodic motion. |
| 2. | To and fro motion of a particle is called oscillatory motion. |
| 3. | Oscillatory motion described in terms of single sine and cosine functions is called simple harmonic motion. |
| 4. | All of the above |
| 1. | \(2\) | 2. | \(1 \over 2\) |
| 3. | Zero | 4. | Infinite |
The rotation of the earth about its axis is:
| 1. | periodic motion. |
| 2. | simple harmonic motion. |
| 3. | periodic and simple harmonic motion. |
| 4. | non-periodic motion. |
All the surfaces are smooth and the system, given below, is oscillating with an amplitude \(\mathrm{A}.\) What is the extension of spring having spring constant \(\mathrm{k_1},\) when the block is at the extreme position?
| 1. | \({k_1 \over k_1+k_2} \text{A}\) | 2. | \({k_2A \over k_1+k_2}\) |
| 3. | \(\mathrm{A}\) | 4. | \(\text{A} \over 2\) |
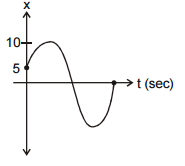
1. \(x= 10\sin\left(\pi t+\frac{\pi}{6}\right)\)
2. \(x= 10\sin\left(\pi t\right)\)
3. \(x= 10\cos\left(\pi t\right)\)
4. \(x= 5\sin\left(\pi t+\frac{\pi}{6}\right)\)
© 2024 GoodEd Technologies Pvt. Ltd.